Math's

Comprehensive Guide to Integration Formula and Techniques

by

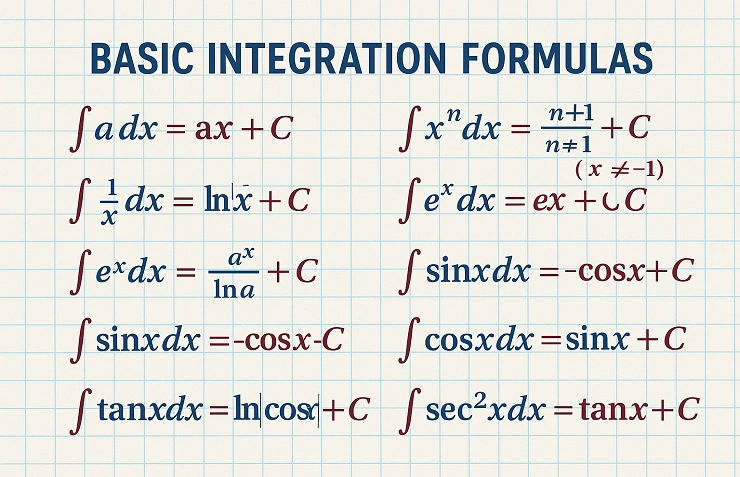
In this you will see integration formula list integration formula list Integration is a fundamental concept in calculus, serving as the reverse process of differentiation. It enables the calculation of areas, volumes, and solutions to differential equations. This article provides an extensive overview of various integration techniques, accompanied by essential formulas and examples.

Basic Integration Formulas

These foundational formulas are crucial for evaluating integrals of elementary functions.

Function Integral
∫1 dx\int 1 \, dx x+Cx + C
∫xn dx\int x^n \, dx xn+1n+1+C\frac{x^{n+1}}{n+1} + C (for n≠−1n \neq -1)
∫sin⁡x dx\int \sin x \, dx −cos⁡x+C-\cos x + C
∫cos⁡x dx\int \cos x \, dx sin⁡x+C\sin x + C
∫sec⁡2x dx\int \sec^2 x \, dx tan⁡x+C\tan x + C
∫csc⁡2x dx\int \csc^2 x \, dx −cot⁡x+C-\cot x + C
∫sec⁡xtan⁡x dx\int \sec x \tan x \, dx sec⁡x+C\sec x + C
∫csc⁡xcot⁡x dx\int \csc x \cot x \, dx −csc⁡x+C-\csc x + C
∫1x dx\int \frac{1}{x} \, dx ( \ln
∫ex dx\int e^x \, dx ex+Ce^x + C
∫ax dx\int a^x \, dx axln⁡a+C\frac{a^x}{\ln a} + C (for a>0,a≠1a > 0, a \neq 1)

These formulas are essential for solving basic integrals in calculus.

Integration of Inverse Trigonometric Functions

Integrals involving inverse trigonometric functions are common in calculus.

Function Integral
∫arcsin⁡x dx\int \arcsin x \, dx xarcsin⁡x+1−x2+Cx \arcsin x + \sqrt{1 – x^2} + C
∫arccos⁡x dx\int \arccos x \, dx xarccos⁡x−1−x2+Cx \arccos x – \sqrt{1 – x^2} + C
∫arctan⁡x dx\int \arctan x \, dx xarctan⁡x−12ln⁡(1+x2)+Cx \arctan x – \frac{1}{2} \ln(1 + x^2) + C
∫\arccotx dx\int \arccot x \, dx x\arccotx+12ln⁡(1+x2)+Cx \arccot x + \frac{1}{2} \ln(1 + x^2) + C
∫\arcsecx dx\int \arcsec x \, dx x\arcsecx−ln⁡(x+x2−1)+Cx \arcsec x – \ln(x + \sqrt{x^2 – 1}) + C
∫\arccscx dx\int \arccsc x \, dx x\arccscx+ln⁡(x+x2−1)+Cx \arccsc x + \ln(x + \sqrt{x^2 – 1}) + C

These integrals are useful in various applications, including physics and engineering.

Integration by Substitution

Also known as uu-substitution, this technique simplifies integrals by changing variables.

Formula:

If u=g(x)u = g(x), then du=g′(x) dxdu = g'(x) \, dx, and

∫f(g(x))g′(x) dx=∫f(u) du\int f(g(x)) g'(x) \, dx = \int f(u) \, du

Example:

Evaluate ∫xcos⁡(x2+1) dx\int x \cos(x^2 + 1) \, dx.

Let u=x2+1u = x^2 + 1, so du=2x dxdu = 2x \, dx. The integral becomes:

12∫cos⁡u du=12sin⁡u+C=12sin⁡(x2+1)+C\frac{1}{2} \int \cos u \, du = \frac{1}{2} \sin u + C = \frac{1}{2} \sin(x^2 + 1) + C

This method is particularly effective for integrals involving composite functions.

Integration By Parts Integration Formula

This technique is based on the product rule for differentiation.

Formula:

∫u dv=uv−∫v du\int u \, dv = uv – \int v \, du

Example:

Evaluate ∫xex dx\int x e^x \, dx.

Let u=xu = x and dv=ex dxdv = e^x \, dx. Then, du=dxdu = dx and v=exv = e^x. Applying the formula:

∫xex dx=xex−∫ex dx=xex−ex+C\int x e^x \, dx = x e^x – \int e^x \, dx = x e^x – e^x + C

This method is useful for products of functions where one function simplifies upon differentiation.

Integration by Partial Fractions

This technique decomposes rational functions into simpler fractions.

Example:

Evaluate ∫1×2−1 dx\int \frac{1}{x^2 – 1} \, dx.

Factor the denominator: x2−1=(x−1)(x+1)x^2 – 1 = (x – 1)(x + 1).

Express the integrand as:

1×2−1=Ax−1+Bx+1\frac{1}{x^2 – 1} = \frac{A}{x – 1} + \frac{B}{x + 1}

Solving for AA and BB, we get:

1×2−1=12(x−1)−12(x+1)\frac{1}{x^2 – 1} = \frac{1}{2(x – 1)} – \frac{1}{2(x + 1)}

Thus, the integral becomes:

∫1×2−1 dx=12ln⁡∣x−1∣−12ln⁡∣x+1∣+C\int \frac{1}{x^2 – 1} \, dx = \frac{1}{2} \ln|x – 1| – \frac{1}{2} \ln|x + 1| + C

This method is effective for rational functions with distinct linear factors.

Trigonometric Integrals

Integrals involving trigonometric functions often require specific techniques.

Examples:

  • ∫sin⁡nx dx\int \sin^n x \, dx and ∫cos⁡nx dx\int \cos^n x \, dx can be evaluated using reduction formulas.

  • ∫sin⁡mxcos⁡nx dx\int \sin^m x \cos^n x \, dx can be evaluated using trigonometric identities and reduction formulas.

These integrals are common in physics, especially in wave and oscillation problems.

Trigonometric Substitutions

This technique is used to simplify integrals involving square roots of quadratic expressions.

Common Substitutions:

  • For a2−x2\sqrt{a^2 – x^2}, use x=asin⁡θx = a \sin \theta.

  • For a2+x2\sqrt{a^2 + x^2}, use x=atan⁡θx = a \tan \theta.

  • For x2−a2\sqrt{x^2 – a^2}, use x=asec⁡θx = a \sec \theta.

These substitutions transform the integrals into trigonometric forms that are easier to evaluate.

Definite Integrals

These integrals compute the area under a curve between two specified points aa and bb.

Formula:

∫abf(x) dx=F(b)−F(a)\int_a^b f(x) \, dx = F(b) – F(a)

where F(x)F(x) is an antiderivative of f(x)f(x).

Function Definite Integral Example
∫0πsin⁡x dx\int_0^\pi \sin x \, dx 22
∫1e1x dx\int_1^e \frac{1}{x} \, dx ln⁡e−ln⁡1=1\ln e – \ln 1 = 1
∫01×2 dx\int_0^1 x^2 \, dx 13\frac{1}{3}

Definite integrals are widely used in applications such as calculating areas, work, and probabilities.

Reduction Formulas

These are recursive formulas that reduce the power of functions in integrals.

Examples:

Function Formula
∫sin⁡nx dx\int \sin^n x \, dx −1nsin⁡n−1xcos⁡x+n−1n∫sin⁡n−2x dx-\frac{1}{n} \sin^{n-1} x \cos x + \frac{n – 1}{n} \int \sin^{n-2} x \, dx
∫xnex dx\int x^n e^x \, dx xnex−n∫xn−1ex dxx^n e^x – n \int x^{n-1} e^x \, dx

These are especially helpful for handling powers of trigonometric and exponential functions.

Advanced Techniques of Integration

Includes techniques such as integration using series expansion, contour integration (in complex analysis), and Laplace transforms (in engineering).

Technique Application
Series Expansion Useful for non-elementary functions
Laplace Transforms Solving differential equations
Complex Integration Used in physics and engineering

These methods are advanced but powerful in tackling complex integrals.

Integration Using Identities

Utilizes mathematical identities to simplify integration.

Examples:

Identity Usage
sin⁡2x=1−cos⁡2×2\sin^2 x = \frac{1 – \cos 2x}{2} Simplifies powers of sine
tan⁡2x=sec⁡2x−1\tan^2 x = \sec^2 x – 1 Reduces expressions involving tangent
ln⁡a−ln⁡b=ln⁡ab\ln a – \ln b = \ln \frac{a}{b} Simplifies logarithmic integrals

This approach is useful when standard forms are not directly applicable.

Integration by Completing the Square

Used for rational functions involving quadratics.

Example:

∫1×2+4x+5 dx\int \frac{1}{x^2 + 4x + 5} \, dx

Complete the square: x2+4x+5=(x+2)2+1x^2 + 4x + 5 = (x + 2)^2 + 1

∫1(x+2)2+1 dx=tan⁡−1(x+2)+C\int \frac{1}{(x + 2)^2 + 1} \, dx = \tan^{-1}(x + 2) + C

Completing the square helps convert expressions to a form involving inverse trigonometric integrals.

Integration Involving Exponentials and Logarithms

These are common in both pure and applied mathematics.

Function Integral
∫eax dx\int e^{ax} \, dx 1aeax+C\frac{1}{a} e^{ax} + C
∫xex dx\int x e^x \, dx xex−ex+Cx e^x – e^x + C
∫ln⁡x dx\int \ln x \, dx xln⁡x−x+Cx \ln x – x + C

These are especially important in solving growth, decay, and differential equation problems.

Special Integrals

These do not have elementary antiderivatives and often involve special functions or numerical methods.

Integral Result
∫e−x2dx\int e^{-x^2} dx Non-elementary, expressed using the error function erf(x)\text{erf}(x)
∫sin⁡xxdx\int \frac{\sin x}{x} dx Non-elementary, known as the Sine Integral Si(x)\text{Si}(x)

Special integrals are essential in advanced analysis and physics.

Beta and Gamma Functions

Used in advanced mathematics and statistics.

Function Definition
Gamma Γ(n)=∫0∞xn−1e−xdx\Gamma(n) = \int_0^\infty x^{n-1} e^{-x} dx, generalizes factorial
Beta B(x,y)=∫01tx−1(1−t)y−1dtB(x, y) = \int_0^1 t^{x-1}(1 – t)^{y-1} dt

These are crucial in evaluating complex integrals in calculus and probability.

Definite Integral Properties

These properties simplify the computation of definite integrals.

Property Formula
Additivity ∫abf(x)dx+∫bcf(x)dx=∫acf(x)dx\int_a^b f(x) dx + \int_b^c f(x) dx = \int_a^c f(x) dx
Reversal of limits ∫abf(x)dx=−∫baf(x)dx\int_a^b f(x) dx = -\int_b^a f(x) dx
Zero width ∫aaf(x)dx=0\int_a^a f(x) dx = 0

Understanding these properties can reduce complex integral evaluations.

Improper Integrals

Used when integration limits approach infinity or the function becomes unbounded.

Example:

∫1∞1x2dx=lim⁡b→∞∫1b1x2dx=1\int_1^\infty \frac{1}{x^2} dx = \lim_{b \to \infty} \int_1^b \frac{1}{x^2} dx = 1

Improper integrals require careful limit evaluation.

Specific and Useful Integration Formulas

Here’s a quick reference list of commonly used integration formulas:

UV Integration Formula

∫u dv=uv−∫v du\int u \, dv = uv – \int v \, du

√(x² + a²) Integration Formula

∫x2+a2 dx=x2x2+a2+a22ln⁡(x+x2+a2)+C\int \sqrt{x^2 + a^2} \, dx = \frac{x}{2} \sqrt{x^2 + a^2} + \frac{a^2}{2} \ln(x + \sqrt{x^2 + a^2}) + C

1/√(a² – x²) Integration Formula

∫1a2−x2dx=arcsin⁡(xa)+C\int \frac{1}{\sqrt{a^2 – x^2}} dx = \arcsin \left( \frac{x}{a} \right) + C

√(a² – x²) Integration Formula

∫a2−x2 dx=x2a2−x2+a22arcsin⁡(xa)+C\int \sqrt{a^2 – x^2} \, dx = \frac{x}{2} \sqrt{a^2 – x^2} + \frac{a^2}{2} \arcsin \left( \frac{x}{a} \right) + C

1/(a² – x²) Integration Formula

∫1a2−x2dx=12aln⁡∣a+xa−x∣+C\int \frac{1}{a^2 – x^2} dx = \frac{1}{2a} \ln \left| \frac{a + x}{a – x} \right| + C

Integration Formula Sheet and Chart

To aid quick reference, it is advisable to maintain an integration formula sheet or chart that summarizes all standard forms and techniques, categorized by:

  • Polynomial

  • Trigonometric

  • Exponential/Logarithmic

  • Inverse Trigonometric

  • Advanced Methods

Would you like me to generate a printable formula sheet or chart as a downloadable PDF or image?

Advanced Integration Techniques 

Integration Using Identities

Mathematical identities can often simplify seemingly complex integrals, particularly in trigonometry integration formula. These identities are especially useful when dealing with powers of trigonometric functions or product-to-sum transformations.

Examples of Trigonometric Identities:

  • Pythagorean Identity:
    sin⁡2x+cos⁡2x=1\sin^2 x + \cos^2 x = 1

  • Double Angle Formulae:
    sin⁡(2x)=2sin⁡xcos⁡x\sin(2x) = 2 \sin x \cos x, cos⁡(2x)=cos⁡2x−sin⁡2x\cos(2x) = \cos^2 x – \sin^2 x

  • Half-Angle Formulae:
    sin⁡2x=1−cos⁡(2x)2\sin^2 x = \frac{1 – \cos(2x)}{2}, cos⁡2x=1+cos⁡(2x)2\cos^2 x = \frac{1 + \cos(2x)}{2}

These identities help simplify integrals, such as:

  • ∫sin⁡2x dx\int \sin^2 x \, dx,

  • ∫cos⁡2x dx\int \cos^2 x \, dx.

Integration by Completing the Square 

Completing the square is an essential method for handling integrals of quadratic forms. It often transforms the integrand into a form where known integral formulas can be applied.

Example:

For ∫1×2+4x+5 dx\int \frac{1}{x^2 + 4x + 5} \, dx, complete the square:

x2+4x+5=(x+2)2+1x^2 + 4x + 5 = (x + 2)^2 + 1

Now, the integral becomes:

∫1(x+2)2+1 dx\int \frac{1}{(x + 2)^2 + 1} \, dx

This is a standard integral with the solution:

tan⁡−1(x+2)+C\tan^{-1}(x + 2) + C

Integration Involving Exponentials and Logarithms 

Exponential and logarithmic functions appear frequently in real-world applications, such as in growth models, decay processes, and differential equations.

Here are some additional integrals involving exponentials and logarithms:

Function Integral
∫eax dx\int e^{ax} \, dx 1aeax+C\frac{1}{a} e^{ax} + C
∫ex2 dx\int e^{x^2} \, dx Non-elementary, requires approximation or series expansion
∫1xln⁡x dx\int \frac{1}{x \ln x} \, dx ln⁡(ln⁡x)+C\ln(\ln x) + C

These integrals form the foundation for many practical problems in science and engineering.

Special Integrals and Functions

Beta and Gamma Functions 

The Beta and Gamma functions are advanced tools used extensively in probability, statistics, and complex analysis.

Function Definition
Gamma Function Γ(n)\Gamma(n) Γ(n)=∫0∞xn−1e−x dx\Gamma(n) = \int_0^\infty x^{n-1} e^{-x} \, dx, extends the factorial function.
Beta Function B(x,y)B(x, y) B(x,y)=∫01tx−1(1−t)y−1 dtB(x, y) = \int_0^1 t^{x-1}(1 – t)^{y-1} \, dt, related to the Gamma function.

The Gamma function, for instance, has the property Γ(n)=(n−1)!\Gamma(n) = (n-1)! for integer values of nn, making it an essential tool in generalizing factorials to non-integer values.

Final Thoughts and Key Takeaways

Integration is a powerful tool in calculus, and mastering the various techniques is crucial for solving complex problems in mathematics, physics, and engineering. Here are the key techniques:

  • Basic Integration Formulas: Essential for quickly evaluating standard integrals.

  • Substitution: Simplifies integrals by changing variables.

  • Integration by Parts: Useful for products of functions.

  • Partial Fractions: Decomposes rational functions into simpler forms.

  • Trigonometric Integrals and Substitutions: Handle integrals involving trigonometric functions and their powers.

  • Definite Integrals: Calculate areas under curves and total accumulated quantities.

  • Advanced Techniques: Include series expansion, Laplace transforms, and the use of special functions such as Beta and Gamma functions.

Integration Formula Chart and Sheet

Here’s a quick summary of the most commonly used formulas:

Integration Formula Result
∫1 dx\int 1 \, dx x+Cx + C
∫xn dx\int x^n \, dx xn+1n+1+C\frac{x^{n+1}}{n+1} + C (for n≠−1n \neq -1)
∫sin⁡x dx\int \sin x \, dx −cos⁡x+C-\cos x + C
∫cos⁡x dx\int \cos x \, dx sin⁡x+C\sin x + C
∫ex dx\int e^x \, dx ex+Ce^x + C
∫1x dx\int \frac{1}{x} \, dx ( \ln
∫1a2−x2 dx\int \frac{1}{\sqrt{a^2 – x^2}} \, dx arcsin⁡(xa)+C\arcsin \left( \frac{x}{a} \right) + C
∫1a2−x2 dx\int \frac{1}{a^2 – x^2} \, dx ( \frac{1}{2a} \ln \left
∫sin⁡2x dx\int \sin^2 x \, dx x2−sin⁡(2x)4+C\frac{x}{2} – \frac{\sin(2x)}{4} + C

Conclusion

Mastering integration involves understanding and applying various techniques and formulas depending on the problem at hand. Whether you’re working with simple polynomials or more advanced functions involving trigonometric, exponential, or logarithmic terms, the key is to choose the right method for simplification. Practice with different techniques, and soon you’ll be able to approach even the most challenging integrals with confidence.

Read Also –Operation Sindoor

Leave a Reply